To reduce a fraction, divide the top and bottom by the highest number that can divide into both numbers exactly. Reducing fractions is also known as simplifying fractions.
Reducing (or simplifying) fractions means to make the fractions as simple as possible.
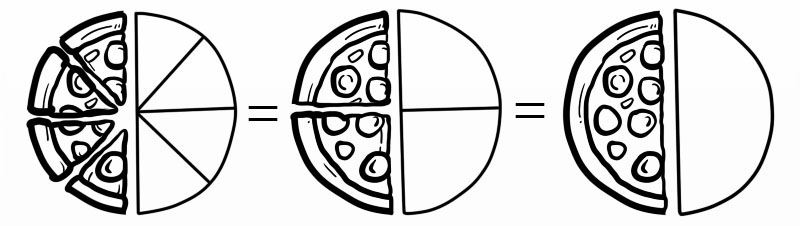
For example, when we say four-eighths (4/8) we really mean (1/2)
There are two ways to simplify a fraction:
Method 1
Try to exactly divide (only whole numbers answers) both the top and bottom of the fraction by 2, 3,5, 7,…..etc, until we can’t go any further.
Example: Reduce the fraction 24/108:
24/108 = 12/54 = 6/27 = 2/9
That is as far as we can go. The fraction simplifies to 2/9.
Example: Reduce the fraction 10/35:
Dividing by 2 does not work because 35 can’t be exactly divided by 2.
Likewise, we can’t divide exactly by 3.
No need to check 4 (we checked 2 already, and 4 is just 2 × 2).
But 5 does work!
10/35 = 2/7
That is far as we can go. The fraction simplifies to 2/7.
Notice that after checking 2 we didn’t need to check 4 (4 = 2×2)
We also don’t need to check 6 when we have checked 2 and 3 (6 is 2×3)
In fact, when checking from smallest to largest we use prime numbers:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37…………
Method 2
Divide the top and bottom of the fraction by the Greatest Common Factor (you have to work it out first!)
Example: Reduce the fraction 8/12:
The largest number that goes exactly into both 8 and 12 is 4, so the Greatest Common Factor is 4.
Divide both top and bottom by 4:
8/12 = 2/3
That is far as we can go. The fraction simplifies to 2/3.